Mathematics has been called “the science of patterns” (Steen, 1988). Young children enjoy working with patterns, and older students enjoy discovering and manipulating patterns. In fact, it is human nature to find patterns in our everyday experiences. Some educators and mathematics would go so far as to say that patterning is the foundation of mathematics (Lee, 1996: Mason 1996). The study of linear growing patterns offers a tangible way for students to think about relationships between quantities. The National Council of Teaching Mathematics (NCTM) recommends that students participate in patterning activities from a young age.
One of the most important reasons for teaching patterning in the younger grades is to help students develop their algebraic thinking (thinking about patterns, variables and relationships). Patterning offers a vehicle for understanding the dependent relations among quantities that underlie mathematical relationships. Working with patterns also offers a way to engage in mathematical thinking that goes beyond “right” and “wrong” answers. Working with patterns can elicit multiple solution strategies from students, allowing them to think about mathematical structure and off justification for their conjectures about the relationships.
Excerpt take from From patterns to algebra: lessons for exploring linear relationships
Ruth Beatty-Catherine Bruce - Nelson Education - 2012
Table and Chairs Problem
Mrs.Chen the principal of Greenvale Public School decided to buy trapezoid shaped tables for the school's new lunch room. While Mrs.Chen waited for the tables to be believed she drew a plan for the lunch room/ She decided that she would place 2 chairs on the long side of each table and 1 chair on every other side. In this way , 5 students could sit around each table. Mrs.Chen found that she could join 2 tables together, and 8 students could sit around them.
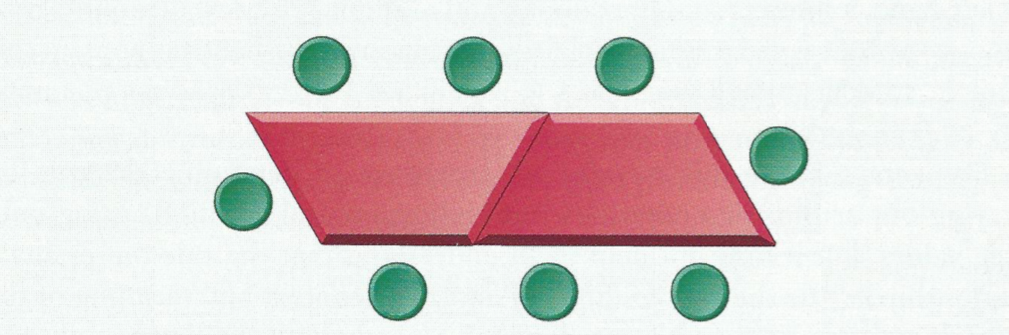
Feel free to use the Pattern Shapes found on Math Learning Center to support your learning!
Primary Task:
How many people could sit around 4 tables?
If we had the same number of tables, but they were shaped as squares/pentagons/octagons (with one chair per side), could we fit more or less people?
Junior Task:
How many chairs would fit around 10 tables?
Create a table to represent the number of people who can sit at the whole table as tables are added on. (e.g., 1 table, 2 tables, 3 tables, 4 tables, etc...)
Intermediate Task:
How many tables would we need for 56 people to be able to sit for lunch?
How many chairs would be able to sit around n number of tables?
Check out the Monthly Math Problem!